A Plane On A Conveyor Belt Is Moving In The Opposite Direction. Can It Take Off?

This page is an appendix to the Physclips page Vectors and relative motion. It was written to answer an odd question put to me by several students. The question was asked with two very different conditions:

Question 1. A plane is on a conveyor belt which, when turned on, moves the plane backwards at a speed equal to the normal take-off speed of the plane. If the pilot uses the normal amount of throttle and other controls…

Can it take off?

Question 2. A plane is on a conveyor belt which, when turned on, moves the plane backwards. The belt speed is controlled and it can move sufficiently fast that, with respect to the ground, the plane never moves forwards. Can the plane take off?

As we shall see, these two sets of conditions are very different. Much of the confusion of the students who have asked this question, and the disagreements among them, can be traced to confusion over which (if either) of these conditions has been specified.

A few assumptions first: Let’s assume that the plane has wheels that roll on the ground, but that there is no engine to drive these wheels. The plane has either propellor or jet engines which push against the air, with negligible effect on the ground. The plane has wings (and other surfaces that we shan’t mention further) that generate lift as air passes over them. We also assume that there is no wind (except later when we consider the wind that may be generated by the conveyor belt itself).

Question 1: belt speed = normal take-off speed. (Technologically, this could be done using an aircraft carrier instead of the conveyor belt, and a plane with a very low take-off speed, such as the Gossamer Condor.) Let’s answer this first by making two approximations, and then deal with the approximations.

Question 2: plane assumed stationary with respect to ground. (This one may not be technologically possible.) This requires looking at some of the same effects, but under very different – and difficult – circumstances.

Question 3: plane assumed stationary with respect to centre of the Earth. (One could do this one experimentally with existing technologies.)

Question 1: belt speed = normal take-off speed

We’ll start by making two approximations, and then consider the effects of these effects more closely.

First we assume that there is no rolling resistance, that the wheels roll completely freely and that they have negligible mass: therefore it takes no force to turn the wheels, even at high speed. This is obviously approximate: because of their mass and thus their moment of inertia, the wheels do require a force (and thus a torque) to make them rotate; because of friction in bearings they require a force (and thus a torque) to keep them rotating; because of the deformation of the tires there is a rolling resistance and this requires a force as well. In principle, however, we could imagine making this effect very small. We might even use hovercraft or maglev instead of wheels.

Air motion. Second, we assume that the conveyor belt does not create a wind with respect to the ground. In practice, the conveyor has to be very long and probably very wide and so will move air to some extent. In principle, however, we could imagine this to be very small: there could be one very narrow belt for each wheel: these would not affect the air much, though it might require precise steering. (On the other hand, if we make the conveyor belt the size of a planet, we end up with Question 3.)

Let’s start the plane’s engines and the conveyor belt simultaneously. The moving conveyor belt starts to rotate the wheels but, according to our initial approximation of freely rotating, massless wheels and no rolling resistance, this requires negligible horizontal force from the ground.

So, subject to our approximations, the horizontal forces on the plane are only the thrust of the engines and the drag exerted by the air. Because we’ve assumed that the conveyor belt doesn’t affect the air, these horizontal forces have their normal values. So the plane accelerates at its normal acceleration and, after a normal period, achieves its take-off velocity and then, with the usual values of flaps etc, takes off. In this case, with these approximations, the question and answer are both simple.

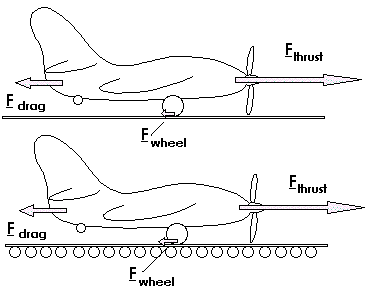
So now let’s look at the approximations. The sketches at right show the horizontal forces at some point during the acceleration towards take-off: the propellor provides forward thrust to the plane. The drag is the plane passes through the air is in the opposite direction. There is also a small backwards force on the wheels.

The runway and the conveyor belt both exert backwards forces on the wheels for three reasons. These effects are all reasonably small compared to the thrust of the engines, but not zero, and not equal for the runway and conveyor cases. What can we say about them?

The wheel bearings are not perfect so, just to keep the wheels turning at constant angular velocity on their axles, a torque is required. This torque is small: give a wheel a spin and observe that its angular velocity decreases due to this effect (and due to air resistance on the tires), but does so slowly. Nevertheless, though small, this is one of the key differences between the plane on the conveyor belt and the plane on the runway. This force increases with angular velocity and, at take-off on the conveyor belt, the wheels must turn twice as fast as on the runway. That’s why, in the sketch, the force on the wheels is very small in the runway case and not quite so small in the conveyor belt case. So, because of this effect alone, one would expect that, in order to take off, the pilot would require very slightly more throttle on the conveyor belt than on the runway.

The wheels also have mass, and therefore a non-zero moment of inertia. (See Rotation, torques, moment of inertia.) So a torque is required for the angular acceleration involved in making them rotate. Lift a bicycle and try spinning the wheel: you have to apply a force to get it spinning. For take-off from the conveyor belt, we have to achieve twice the angular velocity that we’d have on the runway. (Plane is going forward at normal take-off speed, conveyor belt is going backwards at the same speed.) Does this mean twice as much accelerating force? In fact no, because the conveyor belt is like an infinitely long runway: we can take as long as we like (fuel permitting) to spin the wheels up to the required angular velocity. So this is not an important effect in our problem.

Third, there is rolling resistance. The tire is deformed as it rolls, and the energy required to deform it where it touches the ground is a bit less than the energy you recover when it regains its shape after leaving the ground. This gives rise to rolling resistance. Now the amount of deformation of the tire depends on the downwards force. Initially, with the plane stationary with respect to the air, this downwards force on all wheels equals the plane’s weight. As the plane accelerates with respect to the air, however, lift starts to support the weight of the plane, so the downwards force on the wheels becomes successively smaller, and so does the rolling resistance. As we approach take-off speed, rolling resistance becomes very small.

We assumed above that the conveyor belt does not move the air. This can never be quite true, of course. The length of our conveyor belt has to be somewhat longer than the normal take-off distance of the plane. It probably has to be tens of metres wide, too. So it will have an effect on the air above it: it will tend to drag air backwards: it will create a headwind. Pilots usually choose to take off into a headwind: it means that, when they have normal take-off speed with respect to the air, they have a lower speed with respect to the ground. So they achieve take-off speed (with respect to the air) more quickly and need less runway. So, if all other effects were zero, this effect would make it easier for the plane to take off from the conveyor belt than from the runway. The effect, however, is small. (but again, see Question 3 in which this effect dominates.)

So, for question 1, the answer is that the plane will take off at approximately the same speed (with respect to the ground) in the two cases. On the conveyor belt, the take-off speed (with respect to the ground) would be slightly higher due to the wheel bearing effect and slightly lower due to the wind created by the conveyor belt. Which is larger is hard to say, because they can be manipulated by say changing the width of the conveyor belt and forgetting to lubricate the wheel bearings.

Question 2: plane assumed stationary with respect to the ground

Here, the effects discussed above apply, but the assumed condition is different. Here, we are asked to assume that the belt speed is controlled and it can move sufficiently fast that, with respect to the ground, the plane never moves. It’s worth asking whether and how we can do this, because it certainly won’t be easy. Most of those who find this version of the question confusing have not stopped to look at how extreme this condition is. In principle, however, there are two ways.

Wheels. First, we could make the conveyor belt go so fast that the force required to rotate the wheels and the rolling resistance would together be comparable with that exerted by the engine. In principle, at least, this could be done. Normally, these two effects are much smaller than the thrust of the engine. However, if the conveyor belt could go much faster than the normal take-off speed, we could arrange this. It might, however, require a supersonic conveyor belt.

Air motion. Second, if a very large conveyor belt moved backwards at very high speed, it would start to drag air back with it. So the plane would experience a head wind. In principle, we could imagine a case in which the wheel effect were small, and where the conveyor belt moved so quickly that it created a headwind with a speed equal to that of normal take-off. In this (unrealistic) scenario, the plane would be able to take off while stationary with respect to the ground, as sometimes happens in a wind tunnel. Note that this would require a conveyor belt that was both very large and very fast. Getting several hectares of belt moving at hundreds of k.p.h. would also require heroic efforts. (But see Question 3.)

If the first effect were to dominate, then the plane cannot take-off with the normal settings of throttle, flaps etc. If the second dominates, it can. Which is greater? Again, the two effects can be controlled independently. The first effect can be made larger by not lubricating the wheels, or applying the wheel brakes. The second can be made larger with a very large, very fast conveyor belt. So the former is easier to achieve in practice.

However, it’s worth pointing out that the parameters required to achieve the assumed condition would in either case be extraordinary: unless the conveyor belt were extremely large, or unless the wheel brakes were on, the conveyor belt would need to move very much faster than the plane’s take-off speed in order that one of these small effects would achieve the assumed condition. If it were achieved, then whether or not the plane could take off would depend on the relative size of the two effects. (A reader of this page points out that keeping the plane stationary with respect to the ground ‘could be achieved much more simply by reducing the engine’s thrust’. Which is true, of course, but not in the spirit of the question. I doubt that anyone is surprised if a plane doesn’t take off because its engines are only idling.

Question 3: plane assumed stationary with respect to the centre of the Earth

Here’s a more elaborate version, which was suggested by questions from one student, who was interested in what ‘at rest really means’. Suppose, she said, that the plane were initially stationary with respect to an inertial frame at rest with respect to the centre of the earth.

In this frame of reference, the surface of the earth is moving towards the East and, at tropical latitudes, its speed is supersonic. Furthermore, the air is, to a good approximation (complicated by coriolis and thermal effects), moving with the ground (i.e. the surface of the Earth pulls the air along with it, so that local winds are approximately zero, i.e. much slower than the speed due to the Earth’s rotation). So, with respect to both ground and air, a plane at rest in the frame moving with the centre of the Earth is (at normal latitudes) is already travelling Westward at many hundreds of k.p.h. So just choose the latitude that gives the right speed and this hypothetical plane can take vertically (as observed in this frame), with negligible length of runway.

Although this one sounds crazy, one can actually imagine doing it, and it would be techologically easier than Question 2. Take the plane of the question and mount it on top of an airliner (the way NASA does to move the space shuttle). Fly that airliner West at a latitude (probably more than 60° N or S ) at which it can satisfy the condition mentioned of being stationary in an inertial frame at rest with respect to the Earth. Then unbolt the test plane. Viewed from the airliner, it could rise vertically. Viewed from the ground it is still going West at hundreds of k.p.h..

Do NOT forget to Comment Below…